Writing Equations in Slope Intercept Form
Let's first quickly review slope intercept form.
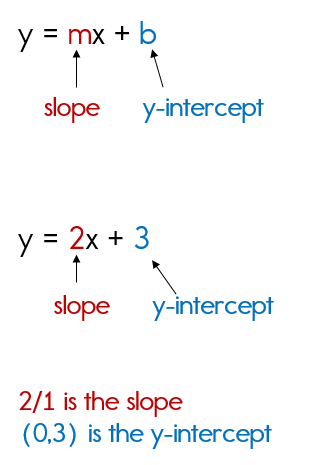
Equations that are written in slope intercept form are the easiest to graph and easiest to write given the proper information.
All you need to know is the slope (rate) and the y-intercept. Continue reading for a couple of examples!
Example 1: Writing an Equation Given the Slope and Y-Intercept
Write the equation for a line that has a slope of -2 and y-intercept of 5.
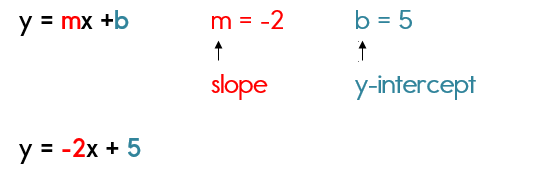
NOTES: I substituted the value for the slope (-2) for m and the value for the y-intercept (5) for b. The variables x and y should always remain variables when writing a linear equation.
In the example above, you were given the slope and y-intercept. Now let's look at a graph and write an equation based on the linear graph.
Example 2: Writing An Equation Based on a Graph
Write an equation that represents the following graph.
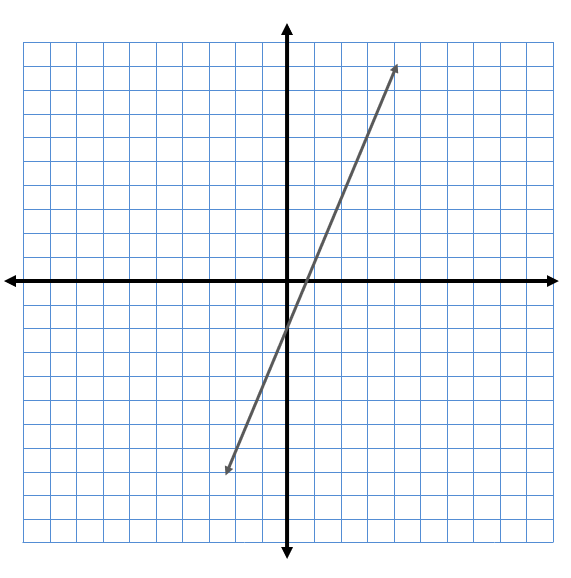
Solution
Step 1: Locate the y-intercept.
Step 2: Locate another point that lies on the line.
Step 3: Calculate the slope from the y-intercept to the second point.
Step 4: Write an equation in slope intercept form given the slope and y-intercept.
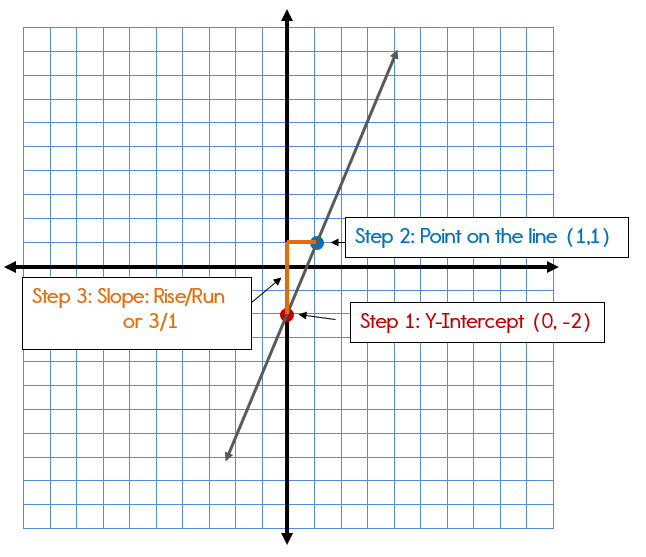
Slope = 3
y-intercept = -2
y = mx + b
y = 3x - 2 is the equation that represents this graph.
Note: You can also check your equation by analyzing the graph. You have a positive slope. Is your graph rising from left to right?
Yes, it is rising; therefore, your slope should be positive!
We've now seen an example of a problem where you are given the slope and y-intercept (Example 1). Example 2 demonstrates how to write an equation based on a graph.
Let's look at one more example where we are given a real world problem. How do we write an equation for a real world problem in slope intercept form?
What will we look for in the problem?
Real World Problems
When you have a real world problem, there are two things that you want to look for!
1. Rate: The rate is your slope in the problem. The following are examples of a rate:
- $3 per day
- $2 an hour
- $5 per person
- $6 a minute
This number is always related to the x value.
"Per" is a key word that is often associated with slope or a rate.
2. A Flat Fee: A flat fee is your y-intercept. This value is a constant or fixed amount. It never changes!
Use the chart below to help you organize your information as you analyze each word problem. This will help you when writing equations
Take a look at the examples below to better clarify how this chart can help you.
Example 3: Writing Equations for Real World Problems
You are visiting Baltimore, MD. A taxi company charges a flat fee of $3.00 plus an additional $0.75 per mile. Write an equation that you could use to find the cost of a taxi ride in Baltimore, MD. Let x represent the number of miles and y represent the total cost.
- How much would a taxi ride for 8 miles cost?
Solution
The y-intercept is 3. Since there is a flat fee of $3, this value becomes the y-intercept. It is a constant, a value that never changes.
The slope is 0.75. This is the rate per mile. A rate is also the slope.
Therefore, the equation that represents this problem is y = 0.75x + 3
- How much would a taxi ride for 8 miles cost?
In order to determine this cost, we will need to use our equation and substitute 8 for x.
y = 0.75x + 3
y = 0.75(8) + 3
9 = (0.75)(8) + 3
The cost of an 8 mile taxi ride is $9.
Hopefully you now have the hang of writing equations in slope intercept form.
Remember to always look for the slope and the y-intercept.
Need More Help With Your Algebra Studies?
Get access to hundreds of video examples and practice problems with your subscription!
Click here for more information on our affordable subscription options.
Not ready to subscribe? Register for our FREE Pre-Algebra Refresher course.
Need Help? Try This Online Calculator!
Affiliate Products...
On this site, I recommend only one product that I use and love and that is Mathway If you make a purchase on this site, I may receive a small commission at no cost to you.
Comments
We would love to hear what you have to say about this page!