Step Functions
Also known as Discontinuous Functions
The graph below is an example of a step function. As you examine the graph, determine why you think it might be called a step function.
Do you see what looks like a set of steps? This is one reason why it is called a step function. It is better known as a discontinuous function.
Why do you think it is called a discontinuous function? Yes, it is not a continuous line, it stops and starts repeatedly.
So, the question may be, is it a function? Does it pass the vertical line test? Let's see!
It looks like the vertical lines may touch two points on the graph at the same time. However, take a look at the points. One is a closed circle and one is an open circle.
If you review our inequalities lesson, you will remember that a closed circle means that the point includes that particular point. But... an open circle does NOT include that point.
So, in this case, where it looks like the vertical line is touching two points, it is really only touching one point, because the open circle does not include that point. So, to answer our question, yes this is considered a function. It's not linear, and it's not quadratic. We call it a step function or a discontinuous function.
How Do We Read and Interpret a Discontinuous Graph?
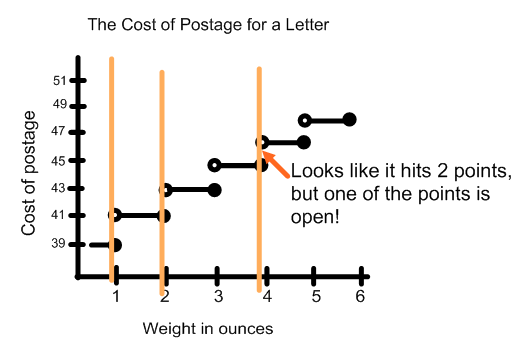
Let's take a look at our postage graph again.
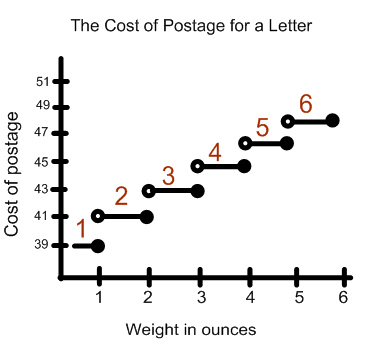
This graph describes how much it will cost to send a letter depending on the weight of the letter. I've labeled the steps so that you better understand the explanation below.
Step 1: If the weight of the letter is over 0 oz and up to 1 oz (including 1 oz, since the circle is closed), it will cost 39 cents.
Step 2: If the weight of the letter is more than 1 oz (not 1 oz exactly because the circle is open) and up to 2 oz (including 2 oz since the circle is closed), then the price is 41 cents.
Step 3: If the weight of the letter is more than 2 oz (not 2 oz exactly because the circle is open) and up to 3 oz (including 3 oz since the circle is closed), then the price is 43 cents.
Steps 4-6 follow the same pattern as steps 1-3 described above.
As you can see, this graph tells you exactly how much your letter will cost depending on the weight. A discontinuous graph must be used because the price stays the same between ounces, but then changes to the next price as you reach a whole ounce.
Let's take a look at a few other discontinuous graphs and determine whether or not they are functions. These graphs may not look like "steps", but they are considered discontinuous.
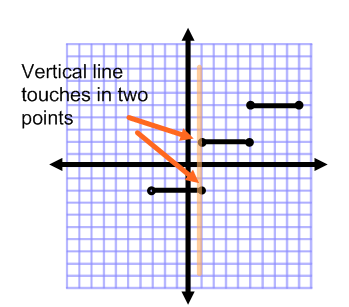
This graph is not a function because when utilizing the vertical line test, it touches in two points.
Both points at x = 1 are solid, therefore the graph is discontinuous, but not a function.
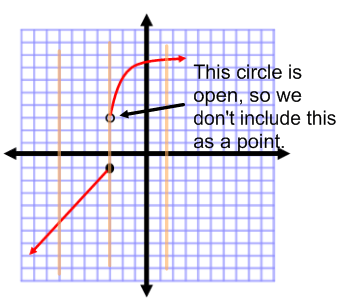
This graph is a function because it passes the vertical line test.
Each vertical line only touches the graph at one point. (Although it looks like it touches at two points at x = -3, since one circle is "open" we do not include that as a point.)
Therefore, it is considered a discontinuous function. It is discontinuous at x = -3.
Let's practice creating and interpreting a graph for a discontinuous function.
Example 1: Step Functions
A wholesale t-shirt manufacturer charges the following prices for t-shirt orders:
- $20 per shirt for shirt orders up to 20 shirts.
- $15 per shirt for shirt between 21 and 40 shirts.
- $10 per shirt for shirt orders between 41 and 80 shirts.
- $5 per shirt for shirt orders over 80 shirts.
- Sketch a graph of this discontinuous function.
- You've ordered 40 shirts and must pay shipping fees of $10. How much is your total order?
Solution
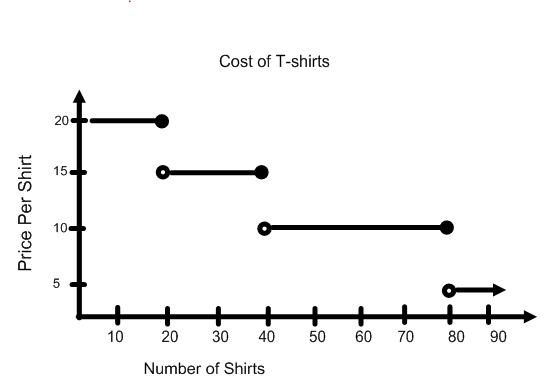
If I ordered 40 shirts and must pay $10 in shipping fees, then my total order will cost $610. (40 * $15) +10 = 610.
Example 2
This would be a great problem for you to try on your own:
In many states a "sales tax" is added to most goods that you buy. The tax rate varies from state to state. Let's suppose that your particular state issues a sales tax on any goods purchased.
You are selling candy bars. The taxable amounts and tax imposed up to $1 are shown below.
For amounts between $0.01 and $0.20, the tax is $.01.
For amounts greater than $0.20 and less than or equal to $0.40, the tax is $0.02.
For amounts greater than $0.40 and less than or equal to $0.60, the tax is $0.03.
For amounts greater than $0.60 and less than or equal to $0.80, the tax is $0.04
For amounts greater than $0.80 and less than or equal to $1.00, the tax is $0.05.
Complete the graph to show the tax that imposed on candy bars.
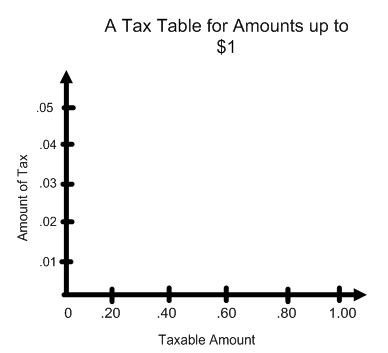
Use the graph to answer the following questions:
1. A candy bar costs $0.55. What is the total cost with tax?
2. Your aunt purchased three candy bars at $0.55 a piece. What is the total cost with tax?
3. Someone purchased 4 candy bars at $0.55 a piece. They gave you $2 and a quarter. Is this enough money to cover the candy bars and the tax? Explain your answer.
Solution
The following is the completed graph for the discontinuous function.
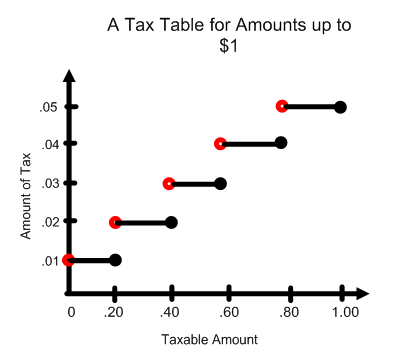
The red circles indicate open circles.
1. If a candy bar costs $0.55, then the total cost with tax is $0.58. (0.55 +0.03)
2. The total cost of three candy bars is $1.74.
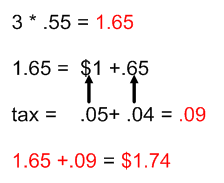
3. If someone gave me $2.25 for 4 candy bars, they would not have given me enough money. The total cost would be $2.31
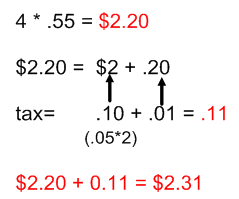
Great Job! You have successfully completed the Step functions lesson.
Need More Help With Your Algebra Studies?
Get access to hundreds of video examples and practice problems with your subscription!
Click here for more information on our affordable subscription options.
Not ready to subscribe? Register for our FREE Pre-Algebra Refresher course.
Need Help? Try This Online Calculator!
Affiliate Products...
On this site, I recommend only one product that I use and love and that is Mathway If you make a purchase on this site, I may receive a small commission at no cost to you.
Comments
We would love to hear what you have to say about this page!