Solving Inequalities in One Variable
Are you ready to dive into our solving inequalities unit? Let's do a very quick review of inequality basics that you probably first learned about in second grade.
Always remember that inequalities do not have just one solution. There are always multiple solutions! Think about the following inequality:
x < 5 (Read as x is less than 5)
- We could replace x with 4 because 4 is less than 5.
- We could replace x with 2 because 2 is less than 5.
- We could even replace x with -3 because -3 is less than 5.
We could go on forever, so as you can see there are many many solutions to this inequality!
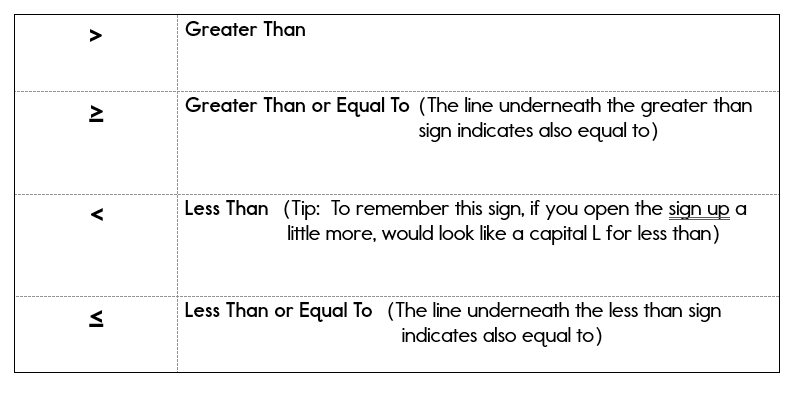
Let's take a look at the inequality symbols and their meanings again.
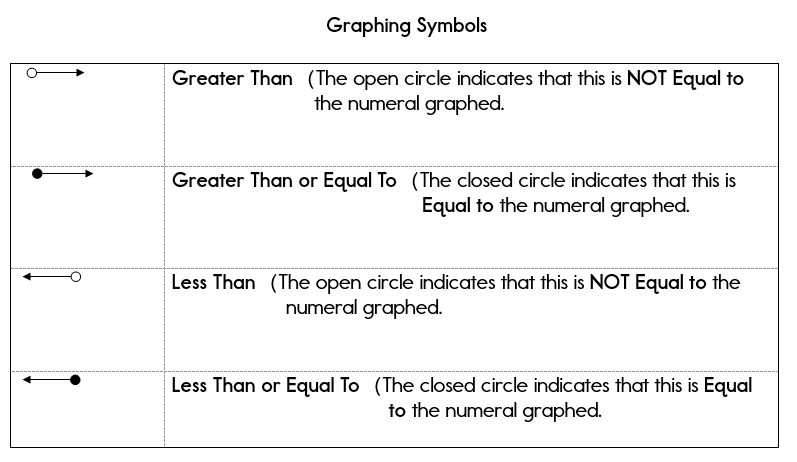
When you graph inequalities that have only one variable, we use a number line. We will use open and closed circles and arrows pointing to the left or right to graph our answers.
An open circle on the graph indicates less than (<) or greater than (>).
A closed circle on the graph represents less than or equal to (<) or greater than or equal to (>).
Take a look at the models below.
x is greater than 5. I use an open circle on 5, since x is NOT equal to 5. Then I draw an arrow to the right to indicate that x can be any number greater than 5.
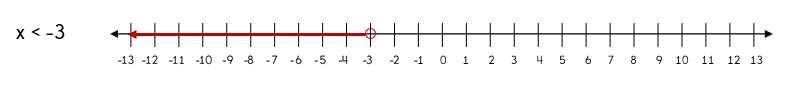
x is less than -3. I use an open circle on -3 since x is NOT equal to -3. Then I draw an arrow to the left to indicate that x can be any number less than -3.
x is greater than OR equal to -2. I use a closed circle on -2 since x is also equal to -2. Then draw an arrow to the right to indicate that x can be any number greater than -2.
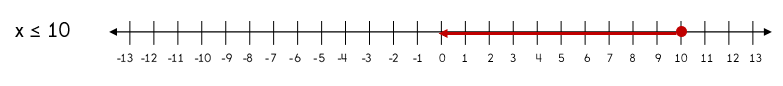
x is less than OR equal to 10. I use a closed circle on 10 since x is also equal to 10. Then draw an arrow to the left to indicate that x can be any number less than 10.
Ok... enough review. Let's solve a few inequalities. You are going to solve inequalities using the exact same rules that you used when solving equations. Let's quickly review those rules:
Rules for Solving Inequalities
- Whatever you do to one side of the inequality, you must do to the other side.
- Always get rid of the constant first and then any coefficients last.
- If you have fractions, get rid of the fractions first and then proceed with solving the inequality.
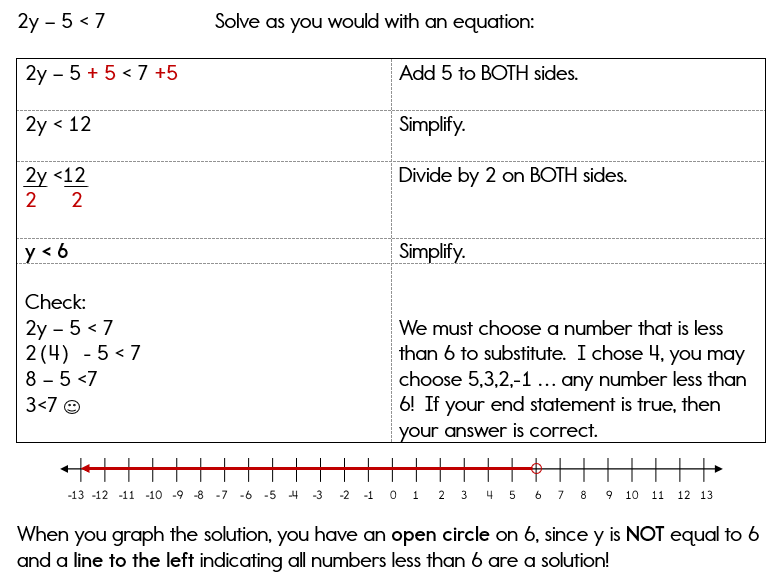
Example 1: Solving an Inequality
Did you notice that you took the exact same steps that you would've taken in order to solve a regular equation? The only difference was that you had a less than sign instead of an equal sign.
Now that was pretty easy!
The next example is similar to example 1, but I would like to show you how to reverse your answer to make it easier to read and graph. Don't be afraid to do this if your variable ends up the right hand side of the inequality.
Example 2: Reversing the Inequality Symbol
Ok... The first two examples should have been pretty easy since you are a superstar at solving equations. Luckily there's only one trick that you have to remember when solving inequalities and that is:
Special Rule for Inequalities
Whenever you multiply or divide by a negative number, you must reverse the sign!
I knew you were going to ask "Why?" And.... you should be asking why. It's important to understand these rules. Sometimes, the best explanations are through examples. Let's take a look.
See What Happens.....
3 < 5 3 is less than 5 is a true statement.
**I know that I can keep my inequality balanced by multiplying or dividing every term by the same number, so.... let's multiply every term by -1.
(-1)(3) < (-1)( 5)
-3 < -5 Now we have -3 is less than -5. Is that a true statement?
No, -3 is greater than -5, so in order to make it a true statement, we must reverse the sign.
-3 > -5
This works with any true statement as long as you multiply or divide by a negative number. Go ahead, try another one. Write a true statement, and then divide by -2.
Ok... let's look at a few examples.
Our next example revisits how to solve equations and/or inequalities with variables on both sides.
Example 3: Variables on Both Sides and Dividing By a Negative Number
Let's take a look at how our new rule applies to solving equations. Pay special attention to the part where we divide by negative 1.
Our last example revisits how to solve equations and/or inequalities with fractions. I hope you remember the trick.
Example 4: Working with Fractions
Now are you ready for a few on your own?
Click here to move onto Solving Inequalities Practice Problems.
Need More Help With Your Algebra Studies?
Get access to hundreds of video examples and practice problems with your subscription!
Click here for more information on our affordable subscription options.
Not ready to subscribe? Register for our FREE Pre-Algebra Refresher course.
Need Help? Try This Online Calculator!
Affiliate Products...
On this site, I recommend only one product that I use and love and that is Mathway If you make a purchase on this site, I may receive a small commission at no cost to you.
Comments
We would love to hear what you have to say about this page!