Probability Lessons:
This lesson focuses on Probability of Compound Events
Thus far, we've studied several probability lessons. If you want to review a few of these lessons before studying compound events, check out the lessons on the fundamental counting principle, independent events, and dependent events.
If you are ready, let's move onto finding the probability of compound events.
Compound events can be further classified as mutually exclusive or mutually inclusive. The probability is calculated differently for each, so let's first take a look at mutually exclusive events.
Compound Events That Are Mutually Exclusive
When two events cannot happen at the same time, they are mutually exclusive events.
For example, you have a die and you are asked to find the probability of rolling a 1 or a 2. You know when you roll the die, only one of those numbers can appear, not both. Therefore, these events are mutually exclusive of each other.
Mutually Exclusive Events (Events that cannot happen at the same time)
P(A or B) = P(A) + P(B)
Take note: With this formula, you are adding the probabilities of each event, not multiplying.
Let's take a look at an example of mutually exclusive events.
Example 1 - Mutually Exclusive Events
Mutually exclusive events are pretty straightforward. Now let's take a look at compound events that are inclusive.
When two events can occur at the same time, they are inclusive.
For example, let's take our example of rolling a regular 6-sided die. You are asked to find the probability of rolling a 2 or an even number.
These events are inclusive because they can happen at the same time. A 2 is an even number, so this would satisfy both, but you could also roll a 4 or 6.
Because 2 is a even number, these are inclusive events.
Inclusive Events (Events that CAN happen at the same time)
P(A or B) = P(A) + P(B) - P(A and B)
This is a little trickier, so let's take a look at example of inclusive events.
Example 2 - Inclusive Events
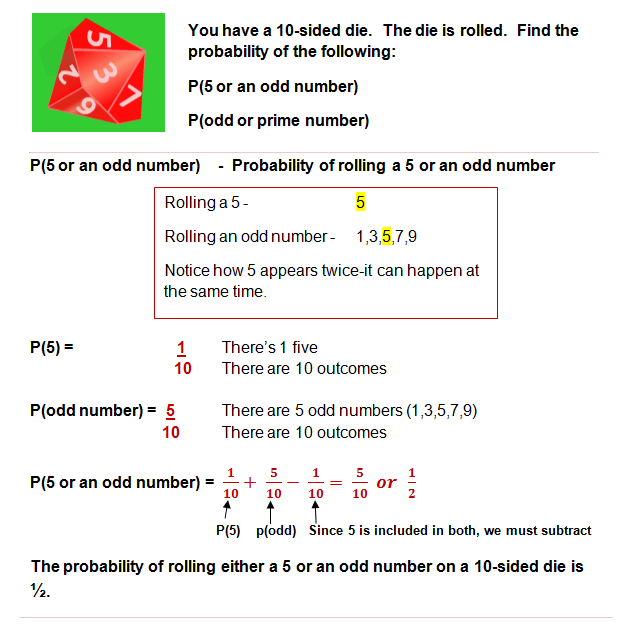
I know that compound events can be confusing, but first you must determine if the events are exclusive or inclusive.
If the events are exclusive, then just add the probabilities of each individual event.
If the events are inclusive, you must remember to subtract the number outcomes that occur in both events.
Your turn to give it a try!
Practice Problems
Directions: First determine if the event is exclusive or inclusive. Then find the probability.
Using a standard deck of cards, find the probability of:
- P(jack or a king)
- P(jack or a spade)
Solutions
So, how are you doing with your probability lessons? I hope you are finding success!
Need More Help With Your Algebra Studies?
Get access to hundreds of video examples and practice problems with your subscription!
Click here for more information on our affordable subscription options.
Not ready to subscribe? Register for our FREE Pre-Algebra Refresher course.
Need Help? Try This Online Calculator!
Affiliate Products...
On this site, I recommend only one product that I use and love and that is Mathway If you make a purchase on this site, I may receive a small commission at no cost to you.
Comments
We would love to hear what you have to say about this page!