Are You Completely Frustrated and In Need of Probability Help?

Have you been searching for probability help, specifically with dependent events? If you are new to Algebra-class.com or just starting a probability unit, you may want to take a look at the introductory probability lesson or the lesson on independent events.

But... if you are ready to study dependent events, let's take a look at the definition.


Dependent Events

Two events, A and B, are dependent if the outcome of the first event does affect the outcome of the second event.

In many cases, the term "without replacement" will be used to signify dependent events.

Dependent Events are notated as:

P(A,then B)

Let's take a closer look at situations with dependent events.


Example 1 - Probability with cards

Probability of dependent events


Did you notice how the playing card was not replaced, so the outcomes and sample space were reduced for the second event?

The second event is dependent on what happens on the first pick. Since this is theoretical probability and we don't know what would really happen on the first pick, we always assume that the first event happens as stated in the problem.

Let's take a look at another example.

Example 2

Probability help with dependent events


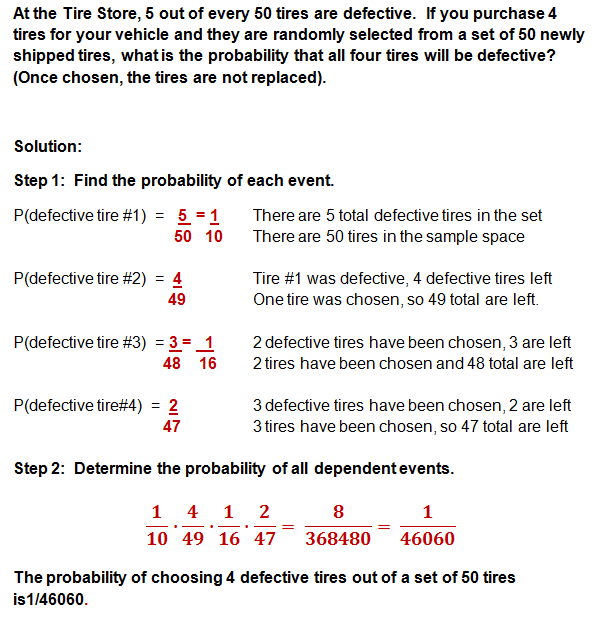
We know that there's not a great chance that ALL 4 tires will be defective, but what are the chances that all four tires will NOT be defective? This is one for you to figure out! Check out the practice problem below.


Practice Problem

At the Tire Store, 5 out of every 50 tires is defective. If you purchase 4 tires for your vehicle and they are randomly selected from a set of 50 newly shipped tires, what is the probability that none of the four tires are defective? (Once chosen, the tires are not replaced)



Solution

Solution to a probability problem

Probability of dependent events is used pretty often in life. You may also like the next lesson on Theoretical Vs. Experimental Probability.




  1. Home
    2. >
  2. Probability
    3. >
  3. Dependent Probability



Comments

We would love to hear what you have to say about this page!

Need More Help With Your Algebra Studies?

Get access to hundreds of video examples and practice problems with your subscription! 

Click here for more information on our affordable subscription options.

Not ready to subscribe?  Register for our FREE Pre-Algebra Refresher course.