Graphing Quadratic Equations

I first introduced the concept of graphing quadratic equations in our Functions unit. In this unit, we discovered how to use a table of values in order to graph a quadratic function. This would be a great lesson to review, as you will see a lot of vocabulary that relates to graphing parabolas.

And... in case you didn't remember, the graph of a quadratic equation is called a parabola. In the following examples, we will pull together all of our knowledge for quadratic equations to create the graph.

Before beginning this lesson, please make sure that you fully understand the vertex formula, factoring quadratic equations, and the quadratic formula.

One way to graph a quadratic equation, is to use a table of values. While this method works for every quadratic equation, there are other methods that are faster.

For any quadratic equation in the form:
y = ax2 + bx + c

The graph will result in a parabola.


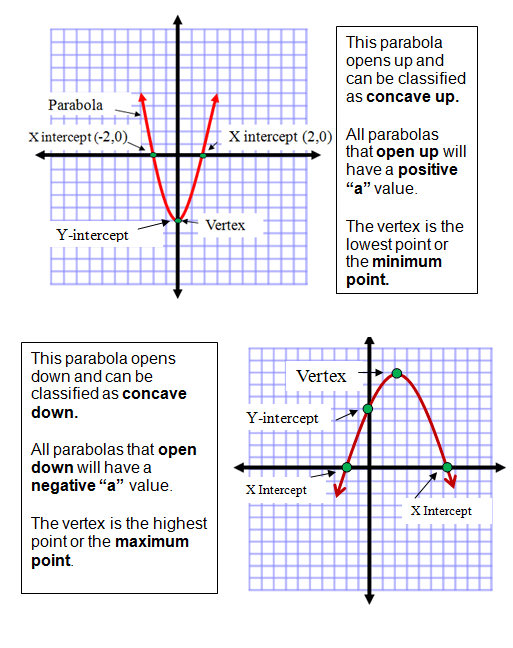
So, how do we find all of these points in order to create the graph?

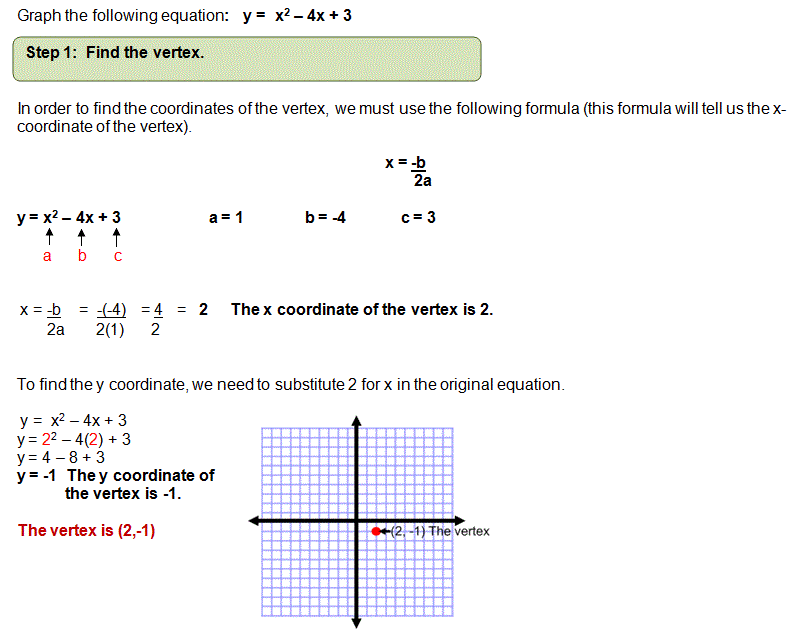
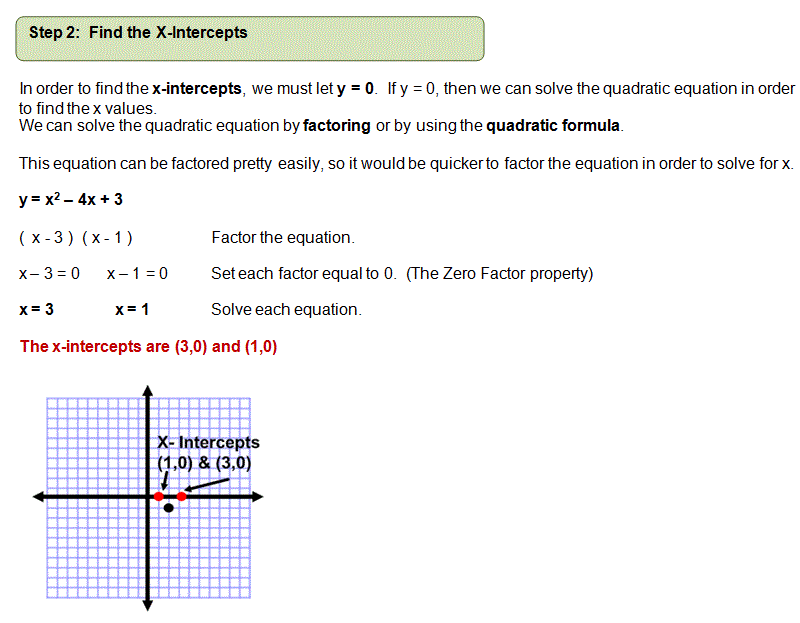
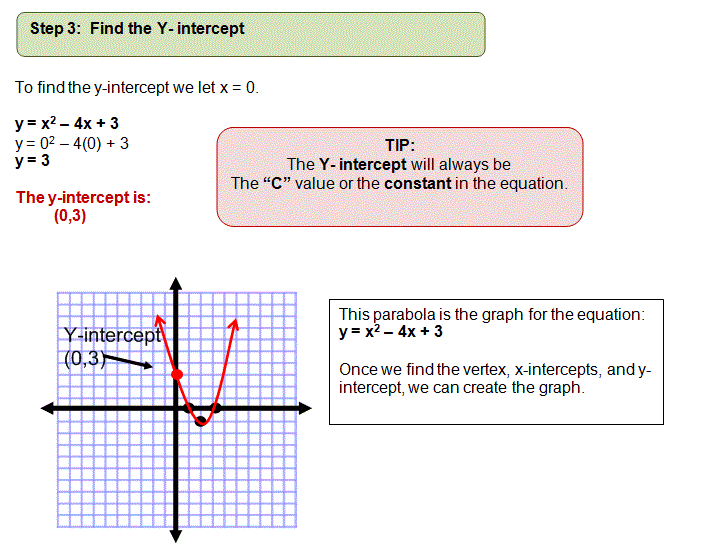
We need to find the vertex, x intercepts, and y intercept. So, let's look at an example and I will show you how to find all the points needed!


Graphing Quadratic Equations - Example 1

Wow! That seems like a lot of work doesn't it? It's really not too bad once you do it a few times.

Did you notice how some of the information that you learned in previous chapters is coming up again? For example, we talked about the vertex formula when we graphed quadratic functions. We also talked about x-intercepts and y-intercepts when we graphed linear equations.

When we graphed linear equations, we let y = 0 when we were trying to find the x-intercepts and we let x = 0 when we were trying to find the y-intercept. We just used the same process for quadratic equations.

This is what I Love about Algebra! You really never forget the concepts because you use them over and over again.

Ok... are you ready to look at one more example? We will graph a quadratic equation that you may not be able to factor as easily. In order to find the x intercepts, we will use the quadratic formula instead of factoring.


Graphing Quadratic Equations - Example 2


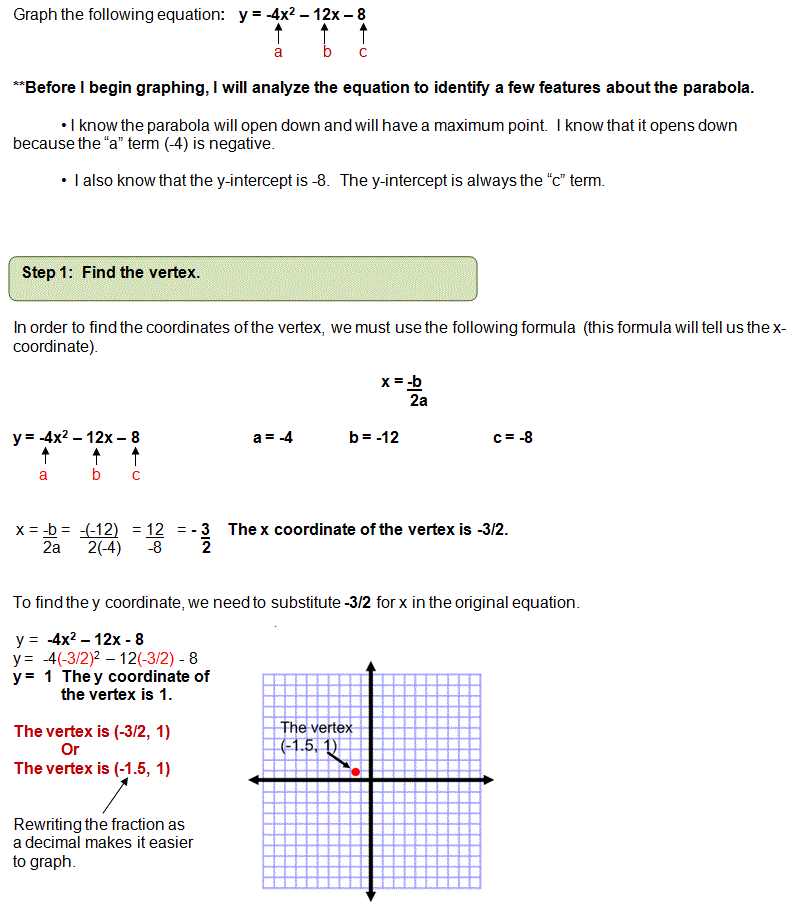
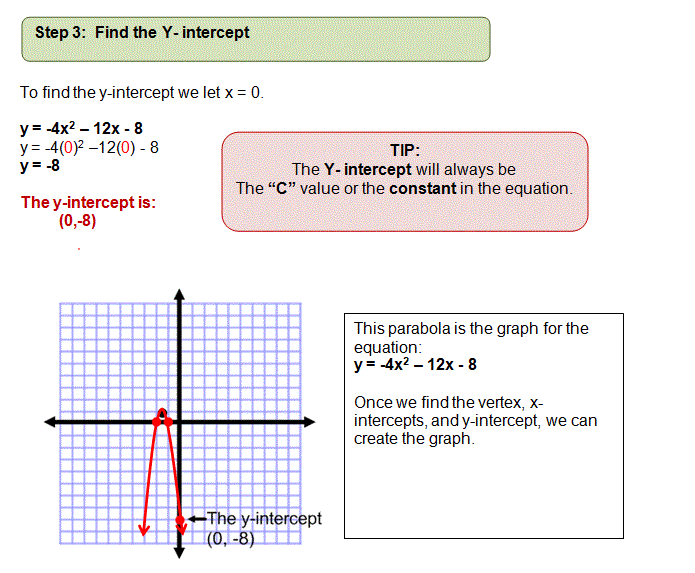
Now I bet you are beginning to understand why factoring is a little faster than using the quadratic formula! It is a lot of work - not too hard, just a little more time consuming.

I hope this helps you to better understand the concept of graphing quadratic equations.


  1. Home
    2. >
  2. Quadratic Equations
    3. >
  3. Graphing Quadratic Equations

Comments

We would love to hear what you have to say about this page!

Need More Help With Your Algebra Studies?

Get access to hundreds of video examples and practice problems with your subscription! 

Click here for more information on our affordable subscription options.

Not ready to subscribe?  Register for our FREE Pre-Algebra Refresher course.