Function Notation

In the previous lesson, you learned how to identify a function by analyzing the domain and range and using the vertical line test.

Now we are going to take a look at function notation and how it is used in Algebra.


The typical notation for a function is f(x). This is read as "f of x" This does NOT mean f times x. This is a special notation used only for functions.

However, f(x) is not the only variable used in function notation. You may see g(x), or h(x), or even b(a). You can use any letters, but they must be in the same format - a variable followed by another variable in parentheses.


FYI

The f(x) function notation was first used by a mathematician named Leonhard Euler in the 1700's.

Often times functions are written as an abbreviation. For example, if you are writing an equation to calculate the square of x. You may write this as a function and name it s(x). This is read as "s of x" for the "square of x".

Another example would be if I were writing an equation to determine the distance a car travels based on a certain time driving. I may write the function as d(t) for "the distance based on the time". This way, I know that t, which represents "time" is my independent variable and d(t) is the outcome.

Ok.. what does this really mean?

Remember when we graphed linear equations? Every equation was written as y = ..... Well, now instead of y = , you are going to see f(x) .....

f(x) is another way of representing the "y" variable in an equation.

Let's take a look at an example.

Examples of function notation

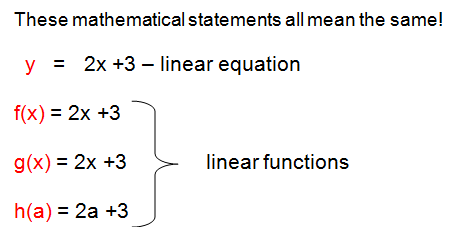
Notice y is replaced with f(x), g(x), even h(a).

This is what is known as function notation. They all mean exactly the same thing. You graph all of these exactly as you would y = 2x +3. We are just using a different notation.

Keep following along with me and you'll learn how to evaluate functions using function notation in the next lesson.

  1. Home
    2. >
  2. Functions
    3. >
  3. Function Notation



Comments

We would love to hear what you have to say about this page!

Need More Help With Your Algebra Studies?

Get access to hundreds of video examples and practice problems with your subscription! 

Click here for more information on our affordable subscription options.

Not ready to subscribe?  Register for our FREE Pre-Algebra Refresher course.