Factoring Quadratic Equations

In my polynomials unit, I demonstrated how to factor trinomials. But, did you ever wonder WHY we need to factor trinomials?

Well, now you are about to find out!

By factoring quadratic equations, we will be able to solve the equation. There are several different ways to solve a quadratic equation. If the equation can be factored, then this method is a quick and easy way to arrive at the solution.

It is EXTREMELY important that you understand how to factor trinomials in order to complete this lesson. If you do not have a thorough understanding of factoring quadratic equations, please review this lesson now!

Let's get started!


We can factor quadratic equations in order to find a solution to the equation because of the Zero-Factor Property.


The Zero-Factor Property

For all real numbers, x and y:

x • y = 0

if and only if

x = 0 or y = 0 (or both)


I know math properties and theorems can be difficult to understand at times. So, the zero-factor property simply means that if the equation is equal to 0, then at least one of the factors must be equal to 0.

Think about it: When you multiply two or more numbers, the only way to get an answer of 0, is if one of the numbers that you are multiplying is 0. That same, very basic property for multiplication applies here.

The key here is that the equation must be set equal to 0.


Let's take a look at an example

Example 1

Notice that all we did to solve was factor the trinomial and then set both factors equal to 0!!

Let's look at another example.

In this example, the given trinomial is not set equal to 0.  When this happens, you must add or subtract to set the trinomial equal to 0.  This must be done before factoring. 

Take a look!

Example 2

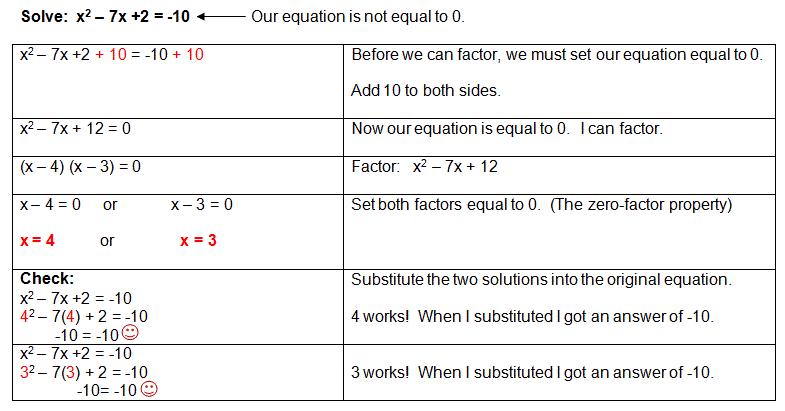
Did you notice how we set the trinomial equal to 0 first by adding 10 to both sides?  Always make sure the trinomial is set equal to 0 before factoring!


Ready to try one on your own?

Solve:    x2 - x - 25 = 5

Answer Key

Notice how our first step is to set the equation equal to 0 by subtracting five from both sides.

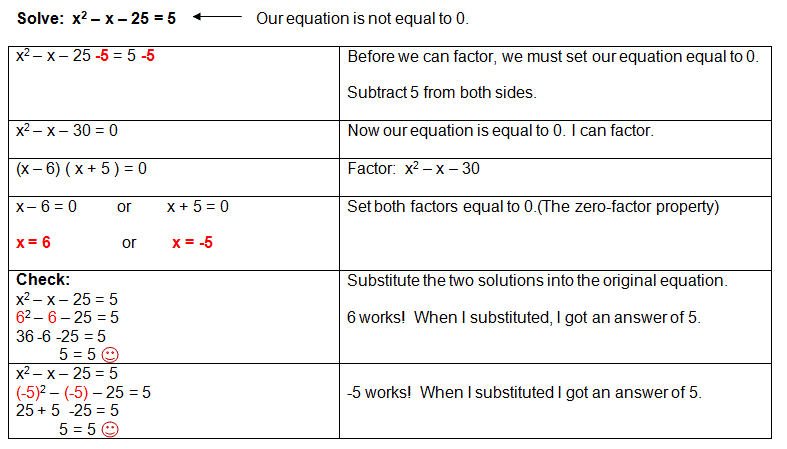
Great job! Now you can move on to solving quadratic equations using the quadratic formula.

Comments

We would love to hear what you have to say about this page!

Need More Help With Your Algebra Studies?

Get access to hundreds of video examples and practice problems with your subscription! 

Click here for more information on our affordable subscription options.

Not ready to subscribe?  Register for our FREE Pre-Algebra Refresher course.