Factoring in Algebra:
Factoring by Grouping

Factoring in Algebra can be accomplished in many different ways. When it comes to polynomials, each situation is different based on the make-up of the polynomial. In our last lesson, we learned how to factor by using the greatest common factor.

However, some polynomials have no greatest common factor other than 1. Therefore, we would need to choose another method for factoring.

In this case, we would look to see if the polynomial has a couple of terms with a common factor. If so, we can group them together and factor separately.

Take a look at the following example:



Example 1: Factoring by Grouping

3x2 - 3 + x2y - y

There are 4 terms in the polynomial. However, there are no common factors within the 4 terms.

Do you see two terms that have a common factor that could be grouped together?


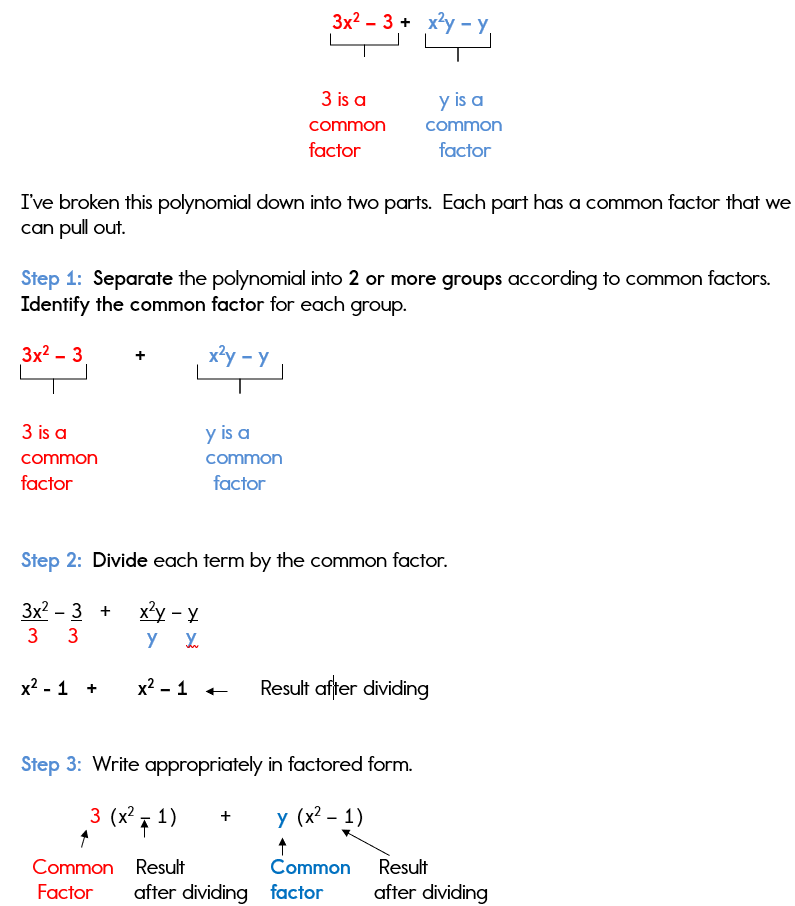
Factoring by grouping
Factoring by grouping 2

I know that factoring can be confusing, but think of factoring as rewriting the problem using the distributive property. You want to continue factoring a polynomial until no common factors exist.

Let's look at another example.


Example 2: Factoring by Grouping

Factoring by Grouping part 1
Factoring by grouping part 2

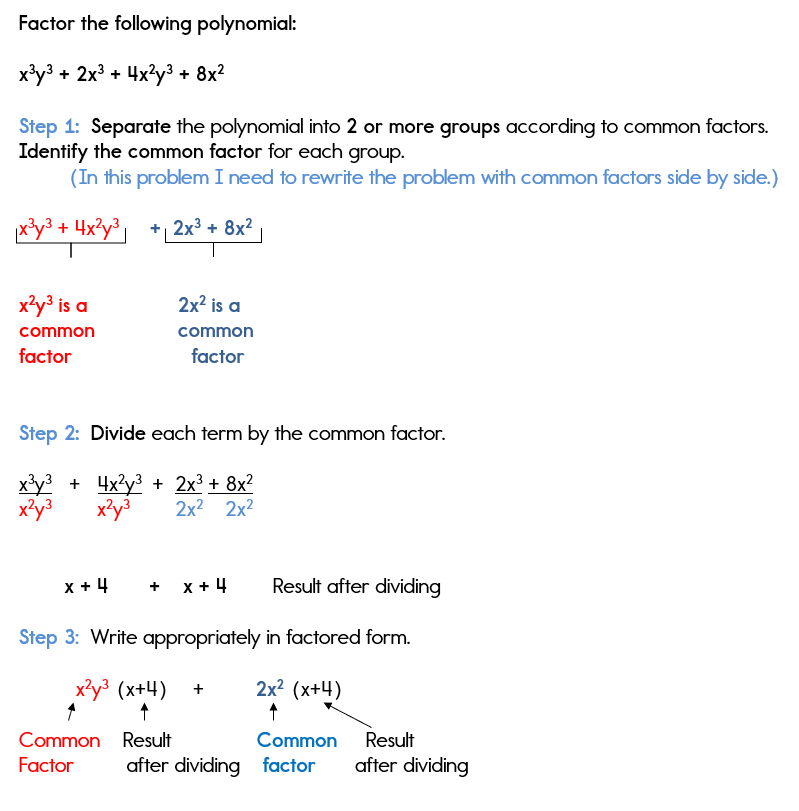
Hopefully you now better understand how to factor polynomials using the grouping method. If you cannot factor by using grouping, then you may have a trinomial that can be factored using a different method.


  1. Home
    2. >
  2. Polynomials
    3. >
  3. Factoring by Grouping



Comments

We would love to hear what you have to say about this page!

Need More Help With Your Algebra Studies?

Get access to hundreds of video examples and practice problems with your subscription! 

Click here for more information on our affordable subscription options.

Not ready to subscribe?  Register for our FREE Pre-Algebra Refresher course.