Solving Compound Inequalities

In our last lesson, we solved compound inequalities that involved the word "and". These are called conjunctions.

In this lesson, we are going to solve the other type of compound inequality that is called a disjunction. These are the compound inequalities that contains the word "or".

There is really only one method to solving disjunction compound inequalities because you must solve each inequality separately.

Let's take a look at an example together.


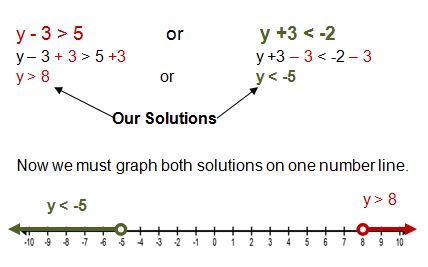
Example 1: Disjunction Compound Inequalities 

Solve and graph: y - 3 > 5 or y +3 < -2

Solving Compound Inequalities

Now do you see why this is called a disjunction? These two inequalities will not have solutions that overlap. You must always solve the two inequalities separately.

Let's take a look at one more example. Remember the rule: If you multiply or divide by a negative number, then you must reverse the inequality sign? Well, that rule still applies. Take a look..


Example 2: Working with Negatives

Solve and graph: -3x - 4 < -7 or -2x +1 > 5


Again, we must solve each inequality separately and then graph the solutions on the same number line.

Solving compound inequalities with negative numbers.

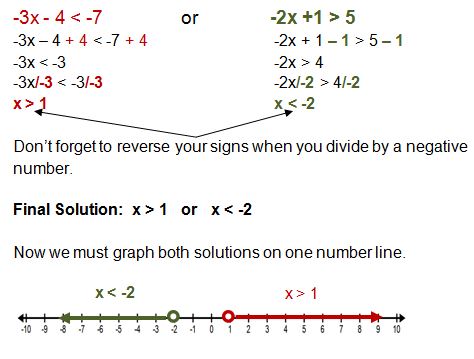
That's our final lesson on compound inequalities. Hopefully you can now tell the difference between a conjunction (using the word "and") and a disjunction (using the word "or"). Check out our other inequality lessons below for a thorough study of inequalities.

  1. Home
    2. >
  2. Inequalities
    3. >
  3. Disjunction Compound Inequalities



Comments

We would love to hear what you have to say about this page!

Need More Help With Your Algebra Studies?

Get access to hundreds of video examples and practice problems with your subscription! 

Click here for more information on our affordable subscription options.

Not ready to subscribe?  Register for our FREE Pre-Algebra Refresher course.